Unit 2 linear functions homework 2 standard and slope-intercept form – Embark on a mathematical odyssey with Unit 2 Linear Functions Homework 2, where we delve into the fundamental concepts of standard and slope-intercept forms. Prepare to unravel the intricacies of linear equations, master the art of graphing, and witness the practical applications of these essential functions in our everyday world.
In this comprehensive guide, we will explore the distinct characteristics of standard and slope-intercept forms, demonstrating their interrelationship and showcasing their advantages and disadvantages. Along the way, we will uncover the secrets of converting between these forms and harness their power to solve real-world problems.
Unit 2: Linear Functions
Linear functions are a fundamental concept in mathematics, with applications in various fields. This unit explores the standard form and slope-intercept form of linear functions, their relationship, and how to graph and solve them.
Standard Form
Standard form, also known as general form, of a linear function is expressed as:
Ax + By = C
where A, B, and C are constants, and A is non-zero. Examples include:
- 2x + 3y = 5
- -4x + 5y = 10
To convert a linear function from other forms into standard form, rearrange the terms to match the standard form equation.
Slope-Intercept Form
Slope-intercept form of a linear function is expressed as:
y = mx + b
where m is the slope and b is the y-intercept. Examples include:
- y = 2x – 1
- y = -3x + 5
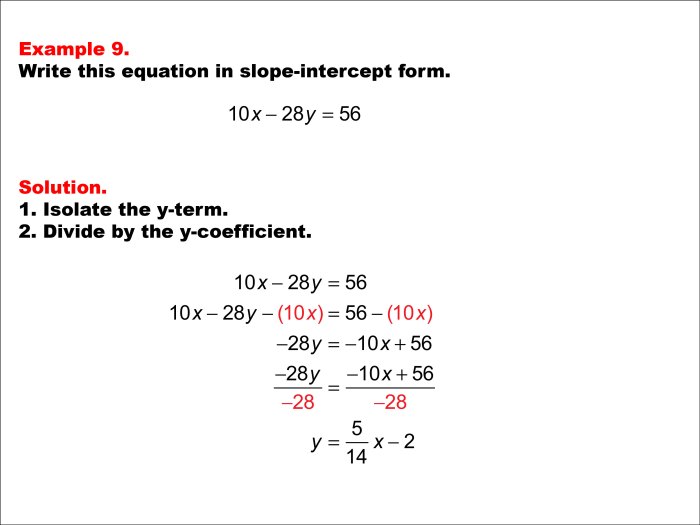
To convert a linear function from other forms into slope-intercept form, solve for y in terms of x.
Relationship Between Standard and Slope-Intercept Forms
Standard form and slope-intercept form are equivalent representations of a linear function. To convert from standard form to slope-intercept form, solve for y:
y = (-A/B)x + (C/B)
To convert from slope-intercept form to standard form, rearrange the equation to:
Ax + By = C
Standard form is useful for finding x- and y-intercepts, while slope-intercept form is convenient for graphing.
Applications of Linear Functions, Unit 2 linear functions homework 2 standard and slope-intercept form
Linear functions have numerous applications, including:
- Modeling linear relationships in real-world scenarios (e.g., population growth, cost-revenue analysis)
- Solving real-world problems involving rates of change, distance, and time
Graphing Linear Functions
To graph a linear function, use the slope and y-intercept. The slope determines the steepness and direction of the line, while the y-intercept is the point where the line crosses the y-axis.
To graph a linear function using the slope and y-intercept:
- Plot the y-intercept on the y-axis.
- Use the slope to determine the direction and steepness of the line.
- Plot additional points and connect them to form the line.
Linear Equations
Linear functions and linear equations are closely related. A linear equation is an equation that can be expressed in the form:
Ax + By = C
where A, B, and C are constants, and A is non-zero. Linear equations can be solved using various methods, including:
- Substitution
- Elimination
- Graphing
FAQ Guide: Unit 2 Linear Functions Homework 2 Standard And Slope-intercept Form
What is the difference between standard form and slope-intercept form?
Standard form (Ax + By = C) emphasizes the coefficients of the variables, while slope-intercept form (y = mx + b) highlights the slope (m) and y-intercept (b).
How do I convert from standard form to slope-intercept form?
Solve the equation for y: y = -Ax/B + C/B
What are the advantages of slope-intercept form?
Slope-intercept form is easy to graph and allows for quick identification of the slope and y-intercept.